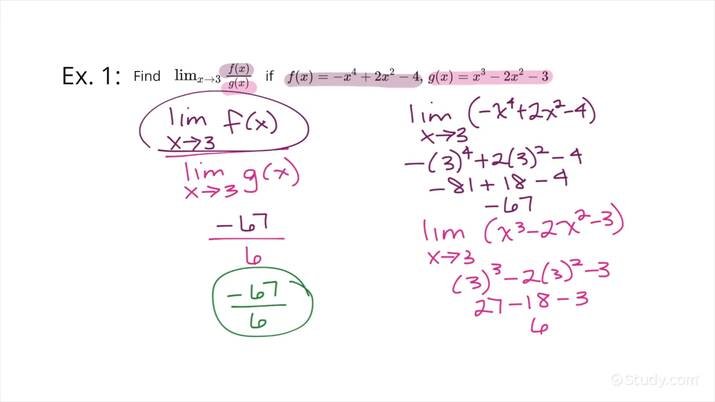In mathematics, limit calculus is a fundamental concept that allows studying the behavior of functions as the given function approaches a specific point. The concept of limit calculus has wide use in finance, physics, engineering, and economics.
The limit is used in other branches of calculus to explain and calculate differential, integral, and continuity of functions. In this article, we’ll explain the definition, rules, and solved examples of limit calculus.
What is Limit Calculus?
In calculus, the limit of a function is a value that a function (f(x)) approaches as the independent variable “x” approaches a particular point. It is denoted by “lim, Lim, or Lt”, and shows the behavior of the function as it gets closer and closer to a specific point.
The mathematical expression of limit calculus is:
Limx→b f(x) = L
The above expression can be read as the function f(x) has a limit “L” at x = b. The epsilon definition of limit calculus is:
The limit of a function will exist at a particular point iff for each positive number ɛ, there must hold a positive number δ, such that
If 0 < |x-a| < δ
Then, |f(x)-L| < ɛ.
Rules of Limit Calculus
Here are some specific rules of limit calculus.
| Rules Name | Rules | Examples |
| Constant Rule | Limx→b [K] = K | Find the limit of 15 at x = 1 Solution Step 1: By constant rule Limx→1 [15] = 15 |
| Power Rule | Limx→b [f(x)]n = [Limx→b f(x)]n | Find the limit of x3 at x = 2 Solution Step 1: Apply the power rule. Limx→2 [x3] = [Limx→2 x]3 Step 2: Apply the Limit value Limx→2 [x3] = [2]3 Limx→2 [x3] = 8 |
| Constant Multiple Rule | Limx→b [K * f(x)] = K * Limx→b f(x) | Find the limit of 4x2 at x = 3 Solution Step 1: Apply the constant function rule. Limx→3 [4x2] = 4Limx→3 [x2] Limx→3 [4x2] = 4[Limx→3 x]2 Step 2: Apply the Limit value Limx→3 [4x2] = 4[3]2 Limx→3 [4x2] = 4[9] Limx→3 [4x2] = 36 |
| Sum Rule | Limx→b [f(x) + g(x)] = Limx→b [f(x)] + Limx→b [g(x)] | Find the limit of 4x2 + 3x3 at x = 3 Solution Step 1: Apply the sum rule. Limx→3 [4x2 + 3x3] = Limx→3 [4x2] + Limx→3 [3x3] Step 2: Apply the constant function rule. Limx→3 [4x2 + 3x3] = Limx→3 4[x2] + 3Limx→3 [x3] Step 3: Apply power rule. Limx→3 [4x2 + 3x3] = 4[Limx→3 x]2 + 3 [Limx→3 x]3 Step 4: Apply Limit value Limx→3 [4x2 + 3x3] = 4[3]2 + 3 [3]3 Limx→3 [4x2 + 3x3] = 4[9] + 3 [27] Limx→3 [4x2 + 3x3] = 36 + 81 Limx→3 [4x2 + 3x3] = 117 |
| Difference Rule | Limx→b [f(x) – g(x)] = Limx→b [f(x)] – Limx→b [g(x)] | Find the limit of 2x3 – 4x at x = 3 Solution Step 1: Apply the difference rule. Limx→3 [2x3 – 4x] = Limx→3 [2x3] – Limx→3 [4x] Step 2: Apply the constant multiple rule. Limx→3 [2x3 – 4x] = 2Limx→3 [x3] – 4Limx→3 [x] Step 3: Apply the power rule. Limx→3 [2x3 – 4x] = 2[Limx→3 x]3 – 4 [Limx→3 x] Step 4: Apply the Limit value Limx→3 [2x3 – 4x] = 2[3]3 – 4 [3] Limx→3 [2x3 – 4x] = 2[27] – 12 Limx→3 [2x3 – 4x] = 54 – 12 Limx→3 [2x3 – 4x] = 42 |
| Product Rule | Limx→b [f(x) * g(x)] = Limx→b [f(x)] * Limx→b [g(x)] | Find the limit of x4 * 2x2 at x = 1 Solution Step 1: Apply the product rule. Limx→1 [x4 * 2x2] = Limx→1 [x4] * Limx→1 [2x2] Step 2: Apply the constant multiple rule. Limx→1 [x4 * 2x2] = Limx→1 [x4] * 2Limx→1 [x2] Step 3: Apply the power rule. Limx→1 [x4 * 2x2] = [Limx→1 x]4 * 2 [Limx→1 x]2 Step 4: Apply the Limit value Limx→1 [x4 * 2x2] = [1]4 * 2 [1]2 Limx→1 [x4 * 2x2] = 1 * 2 (1) Limx→1 [x4 * 2x2] = 1 * 2 Limx→1 [x4 * 2x2] = 2 |
| Quotient Rule | Limx→b [f(x) / g(x)] = Limx→b [f(x)] / Limx→b [g(x)] | Find the limit of 2x4 / 3x2 at x = 2 Solution Step 1: Apply the quotient rule. Limx→2 [2x4 / 3x2] = Limx→2 [2x4] / Limx→2 [3x2] Step 2: Apply the constant multiple rule. Limx→2 [2x4 / 3x2] = 2Limx→2 [x4] / 3Limx→2 [x2] Step 3: Apply the power rule. Limx→2 [2x4 / 3x2] = 2[Limx→2 x]4 / 3 [Limx→2 x]2 Step 4: Apply the Limit value Limx→2 [2x4 / 3x2] = 2[2]4 / 3 [2]2 Limx→2 [2x4 / 3x2] = 2[16] / 3 [4] Limx→2 [2x4 / 3x2] = 32 / 12 Limx→2 [2x4 / 3x2] = 8 / 3 |
Applications of Limit Calculus
Limit calculus has vast applications in mathematics, engineering, and science. Some of the commonly used applications of limit calculus are:
| Applications | Explanation |
| Continuity and Differentiability: | The limits are frequently used to describe continuity and differentiability. The limit of a function is widely used to check whether the function is continuous at a point as well as whether it is differentiable or not. The function is differentiable when it is continuous. |
| Optimization: | Optimization problems are frequently used in limit calculus to evaluate the maximum or minimum values of the functions. Such as the demands and supply can be optimized with the help of limit calculus. |
| Numerical Analysis: | Limits are used in numerical analysis to approximate the values of functions that cannot be computed precisely. For example, the derivative of a function can be approximated using finite differences and limits. |
| Physics and Engineering: | Limits are used comprehensively in physics and engineering to study various physical phenomena, including the behavior of particles, waves, and fluids. For example, limits are used to study the behavior of electric and magnetic fields, and the properties of materials |
| Probability and Statistics: | Limits are used in probability and statistics to study the behavior of random variables and probability distributions. For example, the limit of the sample mean is used to estimate the population means in statistical inference. |
| Computer Science: | Limits are used in computer science to analyze the performance of algorithms and programs. For example, the limit of the running time of an algorithm is used to determine its computational complexity. |
How to calculate limit problems?
Here is an example to learn how to evaluate the limit problems.
Example
Find the limit of 5x2 + 3x – 12 * 3x3 / 3x2 when x approaches 3.
Solution
Step 1: Take the given algebraic expression and write it according to the general expression of limit calculus.
Limx→3 [5x2 + 3x – 12 * 3x3 / 3x2]
Step 2: Now apply the sum, difference, product, and quotient rules of limit calculus to the above algebraic expression.
Limx→3 [5x2 + 3x – 12 * 3x3 / 3x2] = Limx→3 [5x2] + Limx→3 [3x] – Limx→3 [12] * Limx→3 [3x3] / Limx→3 [3x2]
Step 3: Now apply the constant multiple rules to the above expression.
Limx→3 [5x2 + 3x – 12 * 3x3 / 3x2] = 5Limx→3 [x2] + 3Limx→3 [x] – Limx→3 [12] * 3Limx→3 [x3] / 3Limx→3 [x2]
Step 4: Now apply the power rule and constant rules.
Limx→3 [5x2 + 3x – 12 * 3x3 / 3x2] = 5 [Limx→3 x]2 + 3 [Limx→3 x] – Limx→3 [12] * 3 [Limx→3 x]3 / 3 [Limx→3 x]2
Step 5: Apply Limit value x = 3
= 5 [3]2 + 3 [3] – [12] * 3 [3]3 / 3 [3]2
= 5 [9] + 3 [3] – [12] * 3 [27] / 3 [9]
= 45 + 9 – 12 * 81 / 27
= 45 + 9 – 972 / 27
= 45 + 9 – 36
= 18
Conclusion
The limit is the fundamental concept in calculus to deal with the behavior of the functions at a particular point.
The rules of the limit are very essential to evaluate the limit of the function. The applications of limit calculus are very vast.